| The transformations you have seen in the past can also be used to move and resize graphs of functions. We will be examining the following changes to f (x):
- f (x), f (-x),f (x) + k, f (x + k), kf (x), f (kx)
reflections translations dilations | Reflections of Functions: -f (x) and f (-x) | |  Reflection over the x-axis.
-f (x) reflects f (x) over the x-axis
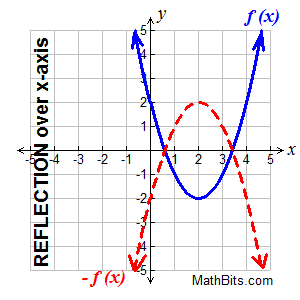|  | Vertical Reflection:
Reflections are mirror images. Think of "folding" the graph over the x-axis. | On a grid, you used the formula
(x,y) → (x,-y) for a reflection in the
x-axis, where the y-values were negated. Keeping in mind that y = f (x),
we can write this formula as
(x, f (x)) → (x, -f (x)). |  |
| | Reflection over the y-axis.
f (-x) reflects f (x) over the y-axis | 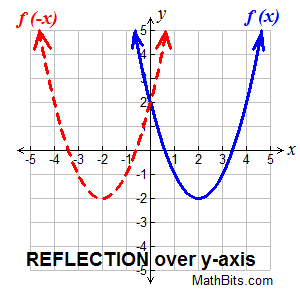 | | Horizontal Reflection:
Reflections are mirror images. Think of "folding" the graph over the y-axis. On a grid, you used the formula (x,y) → (-x,y) for a reflection in the y-axis, where the x-values were negated. Keeping in mind that
y = f (x), we can write this formula as
(x, f (x)) → (-x, f (-x)). 
| | Translations of Functions: f (x) + k and f (x + k) | | Translation vertically (upward or downward)
f (x) + k translates f (x) up or down | 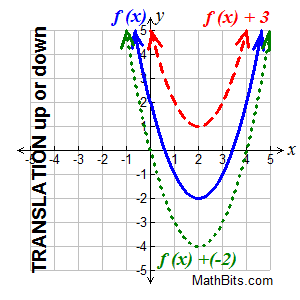 Changes occur "outside" the function
(affecting the y-values). | Vertical Shift:
This translation is a "slide" straight up or down.
• if k > 0, the graph translates upward k units.
• if k < 0, the graph translates downward k units. On a grid, you used the formula (x,y) → (x,y + k) to move a figure upward or downward. Keeping in | mind that y = f (x), we can write this formula as (x, f (x)) → (x, f (x) + k ).
Remember, you are adding the value
of k to the y-values of the function. |  | | | Translation horizontally (left or right)
f (x + k) translates f (x) left or right |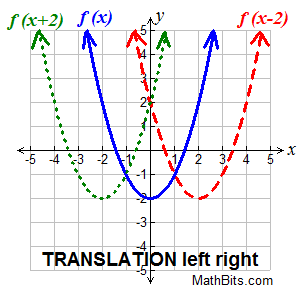 
Changes occur "inside" the function
(affecting the x-axis). | | Horizontal Shift:
This translation is a "slide" left or right.
• if k > 0, the graph translates to the left k units.
• if k < 0, the graph translates to the right k units. 
This one will not be obvious from the patterns you previously used when translating points.
k positive moves graph left
k negative moves graph right
A horizontal shift means that every point (x,y) on the graph of f (x) is transformed to (x - k, y) or (x + k, y) on the graphs of y = f (x + k) or y = f (x - k) respectively.
Look carefully as this can be very confusing!
| | Hint: To remember which way to move the graph, set (x + k) = 0. The solution will tell you in which direction to move and by how much.
f (x - 2): x - 2 = 0 gives x = +2, move right 2 units.
f (x + 3): x + 3 = 0 gives x = -3, move left 3 units. |  | | Up to this point, we have only changed the "position" of the graph of the function.
Now, we will start changing "distorting" the shape of the graphs. | Dilations of Functions: kf (x) and f (kx) | | Vertical Stretch or Compression (Shrink)
k f (x) stretches/shrinks f (x) vertically |  "Multiply y-coordinates"
(x, y) becomes (x, ky)
"vertical dilation" | | A vertical stretching is the stretching of the graph away from the x-axis
A vertical compression (or shrinking) is the squeezing of the graph toward the x-axis.
• if k > 1 , the graph of y = k•f (x) is the graph of f (x) vertically stretched by multiplying each of its y-coordinates by k.
• if 0 < k < 1 (a fraction), the graph is f (x) vertically shrunk (or compressed) by multiplying each of its y-coordinates by k.
| • if k should be negative, the vertical stretch or shrink is followed by a reflection across the x-axis.
Notice that the "roots" on the graph stay in their same positions on the x-axis. The graph gets "taffy pulled" up and down from the locking root positions. The y-values change. |  | | | Horizontal Stretch or Compression (Shrink)
f (kx) stretches/shrinks f (x) horizontally | 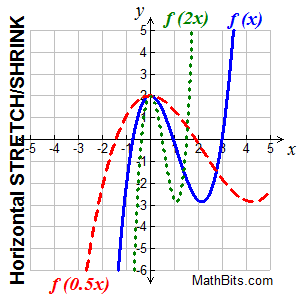 "Divide x-coordinates"
(x, y) becomes (x/k, y)
"horizontal dilation" | | A horizontal stretching is the stretching of the graph away from the y-axis
A horizontal compression (or shrinking) is the squeezing of the graph toward the y-axis.
• if k > 1 , the graph of y = f (k•x) is the graph of f (x) horizontally shrunk (or compressed) by dividing each of its x-coordinates by k.
• if 0 < k < 1 (a fraction), the graph is f (x) horizontally stretched by dividing each of its x-coordinates by k.
• if k should be negative, the horizontal stretch or shrink is followed by a reflection in the y-axis.
| Notice that the "roots" on the graph have now moved, but the y-intercept stays in its same initial position for all graphs. The graph gets "taffy pulled" left and right from the locking y-intercept. The x-values change. |  | | | Transformations of Function Graphs | | | reflect f (x) over the x-axis | | f (-x) | reflect f (x) over the y-axis | | f (x) + k | shift f (x) up k units | | f (x) - k | shift f (x) down k units | | f (x + k) | shift f (x) left k units | | f (x - k) | shift f (x) right k units | | k•f (x) | multiply y-values by k (k > 1 stretch, 0 < k < 1 shrink vertical) | | f (kx) | divide x-values by k(k > 1 shrink, 0 < k < 1 stretch horizontal) |  NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
0 Response to "explain how to use a graph of the function f(x) to find f(3)."
Post a Comment